Difference between revisions of "Lecture 11"
Jump to navigation
Jump to search

| (One intermediate revision by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | <div style="padding: 5px; background: #FF4560; border:solid 2px #000000;"> | ||
| + | '''Update Warning!''' | ||
| + | This page has not been revised yet for the 2007 Fall term. Some of the slides may be reused, but please consider the page as a whole out of date as long as this warning appears here. | ||
| + | </div> | ||
| + | | ||
| + | |||
| + | | ||
| + | |||
| + | |||
__NOTOC__ | __NOTOC__ | ||
<small>[[Lecture_10|(Previous lecture)]] ... [[Lecture_12|(Next lecture)]]</small> | <small>[[Lecture_10|(Previous lecture)]] ... [[Lecture_12|(Next lecture)]]</small> | ||
| Line 127: | Line 136: | ||
======Slide 027====== | ======Slide 027====== | ||
[[Image:L11_s027.jpg|frame|none|Lecture 11, Slide 027<br> | [[Image:L11_s027.jpg|frame|none|Lecture 11, Slide 027<br> | ||
| + | Natural proteins of course have evolved under the constraint of foldability. They may have avoided mutations that would expose them to the requirements of full, combinatorial optimization of their 3-D structure. | ||
| + | ]] | ||
| − | |||
======Slide 028====== | ======Slide 028====== | ||
[[Image:L11_s028.jpg|frame|none|Lecture 11, Slide 028<br> | [[Image:L11_s028.jpg|frame|none|Lecture 11, Slide 028<br> | ||
Latest revision as of 15:27, 1 September 2007
Update Warning! This page has not been revised yet for the 2007 Fall term. Some of the slides may be reused, but please consider the page as a whole out of date as long as this warning appears here.
(Previous lecture) ... (Next lecture)
Protein Structure Prediction
...
Add:
- Summary points
- Exercises
- Further reading
Lecture Slides
Slide 001
Slide 002
Slide 003
Slide 004
Slide 005
Slide 006
Slide 007
Slide 008
Slide 009
Slide 010
Slide 011
Slide 012
Slide 013
Slide 014
Slide 015
Slide 016
Slide 017
Slide 018

Lecture 11, Slide 018
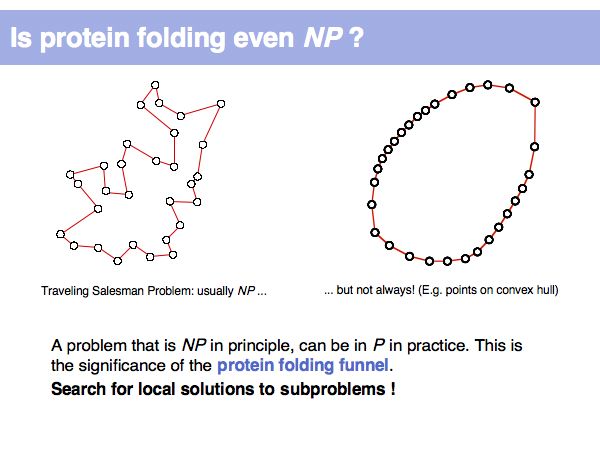
Non-polynomial time-complexity problems are considered intractable, since even as the problem size 'n' grows only modestly, the time requirements grow beyond all bounds and reasonable resources. A 1,000 element problem of O(2n) complexity takes the age of the universe to compute.
Non-polynomial time-complexity problems are considered intractable, since even as the problem size 'n' grows only modestly, the time requirements grow beyond all bounds and reasonable resources. A 1,000 element problem of O(2n) complexity takes the age of the universe to compute.